How To Find The Exponential Function Given Two Points

Learning Outcomes

• Given 2 data points, write an exponential role.
• Identify initial conditions for an exponential function.
• Find an exponential function given a graph.
• Utilize a graphing calculator to find an exponential part.
• Find an exponential function that models continuous growth or decay.

In the previous examples, we were given an exponential part which we then evaluated for a given input. Sometimes we are given data about an exponential office without knowing the part explicitly. We must apply the information to first write the form of the function, decide the constants a and b, and evaluate the function.

How To: Given two data points, write an exponential model

1. If ane of the data points has the form [latex]\left(0,a\right)[/latex], then a is the initial value. Using a, substitute the second bespeak into the equation [latex]f\left(x\right)=a{b}^{x}[/latex], and solve for b.
2. If neither of the data points have the grade [latex]\left(0,a\right)[/latex], substitute both points into ii equations with the form [latex]f\left(x\right)=a{b}^{x}[/latex]. Solve the resulting arrangement of two equations to find [latex]a[/latex] and [latex]b[/latex].
3. Using the a and b found in the steps above, write the exponential role in the class [latex]f\left(ten\correct)=a{b}^{10}[/latex].

Case: Writing an Exponential Model When the Initial Value Is Known

In 2006, lxxx deer were introduced into a wildlife refuge. By 2012, the population had grown to 180 deer. The population was growing exponentially. Write an algebraic function N(t) representing the population N of deer over time t.

Try It

A wolf population is growing exponentially. In 2011, 129 wolves were counted. By 2013 the population had reached 236 wolves. What two points tin exist used to derive an exponential equation modeling this situation? Write the equation representing the population North of wolves over fourth dimension t.

Show Solution

[latex]\left(0,129\right)[/latex] and [latex]\left(2,236\right);N\left(t\right)=129{\left(\text{one}\text{.3526}\right)}^{t}[/latex]

Example: Writing an Exponential Model When the Initial Value is Not Known

Find an exponential role that passes through the points [latex]\left(-2,6\right)[/latex] and [latex]\left(2,1\correct)[/latex].

Endeavor It

Given the two points [latex]\left(ane,3\right)[/latex] and [latex]\left(2,iv.five\right)[/latex], find the equation of the exponential office that passes through these two points.

Show Solution

[latex]f\left(x\right)=two{\left(1.v\right)}^{ten}[/latex]

Q & A

Practice two points always determine a unique exponential function?

Yes, provided the two points are either both above the x-centrality or both below the x-centrality and have dissimilar x-coordinates. Simply keep in heed that we also need to know that the graph is, in fact, an exponential office. Not every graph that looks exponential really is exponential. We need to know the graph is based on a model that shows the same percent growth with each unit of measurement increase in ten, which in many real world cases involves time.

How To: Given the graph of an exponential function, write its equation

1. First, identify two points on the graph. Cull the y-intercept every bit one of the 2 points whenever possible. Effort to cull points that are as far apart as possible to reduce circular-off mistake.
2. If ane of the data points is the y-intercept [latex]\left(0,a\correct)[/latex] , then a is the initial value. Using a, substitute the second indicate into the equation [latex]f\left(x\right)=a{b}^{10}[/latex] and solve for b.
3. If neither of the data points accept the course [latex]\left(0,a\right)[/latex], substitute both points into two equations with the form [latex]f\left(ten\right)=a{b}^{x}[/latex]. Solve the resulting system of ii equations to find a and b.
4. Write the exponential function, [latex]f\left(ten\right)=a{b}^{x}[/latex].

Example: Writing an Exponential Function Given Its Graph

Find an equation for the exponential function graphed below.

Endeavor It

Find an equation for the exponential role graphed below.

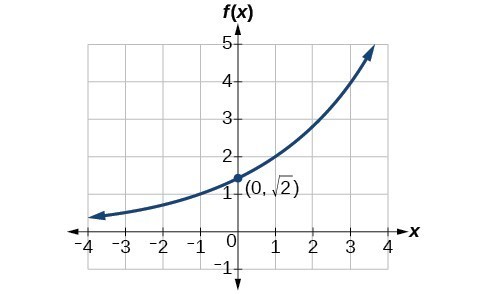
Prove Solution

[latex]f\left(x\right)=\sqrt{2}{\left(\sqrt{2}\right)}^{x}[/latex]. Answers may vary due to round-off error. The answer should be very shut to [latex]i.4142{\left(1.4142\right)}^{x}[/latex].

Investigating Continuous Growth

So far we accept worked with rational bases for exponential functions. For nigh real-earth phenomena, even so, e is used as the base for exponential functions. Exponential models that use e every bit the base are called continuous growth or disuse models. We see these models in finance, computer science, and nearly of the sciences such every bit physics, toxicology, and fluid dynamics.

A General Note: The Continuous Growth/Decay Formula

For all existent numbers t, and all positive numbers a and r, continuous growth or decay is represented by the formula

[latex]A\left(t\correct)=a{e}^{rt}[/latex]

where

• a is the initial value
• r is the continuous growth rate per unit of time
• t is the elapsed time

If r> 0, and then the formula represents continuous growth. If r< 0, then the formula represents continuous disuse.

For business applications, the continuous growth formula is called the continuous compounding formula and takes the grade

[latex]A\left(t\right)=P{eastward}^{rt}[/latex]

where

• P is the principal or the initial investment
• r is the growth or involvement charge per unit per unit of time
• t is the menses or term of the investment

How To: Given the initial value, rate of growth or decay, and time t, solve a continuous growth or decay function

1. Use the information in the problem to make up one's mind a, the initial value of the function.
2. Use the information in the problem to determine the growth charge per unit r.
   • If the problem refers to continuous growth, and then r> 0.
   • If the problem refers to continuous disuse, and so r< 0.
3. Use the information in the problem to determine the fourth dimension t.
4. Substitute the given information into the continuous growth formula and solve for A(t).

Example: Calculating Continuous Growth

A person invested $one,000 in an account earning a nominal involvement rate of ten% per yr compounded continuously. How much was in the business relationship at the stop of i twelvemonth?

Try It

A person invests $100,000 at a nominal 12% interest per yr compounded continuously. What will be the value of the investment in xxx years?

Evidence Solution

$three,659,823.44

Instance: Computing Continuous Decay

Radon-222 decays at a continuous rate of 17.3% per mean solar day. How much will 100 mg of Radon-222 disuse to in 3 days?

Attempt Information technology

Using the information in the previous case, how much radon-222 will remain later on one year?

Prove Solution

three.77E-26 (This is calculator notation for the number written as [latex]3.77\times {10}^{-26}[/latex] in scientific annotation. While the output of an exponential function is never zero, this number is so shut to aught that for all practical purposes nosotros tin can accept cipher as the reply.)

Contribute!

Did you have an idea for improving this content? We'd dearest your input.

Improve this pageLearn More

Source: https://courses.lumenlearning.com/waymakercollegealgebra/chapter/find-the-equation-of-an-exponential-function/

Posted by: rettigthedidismind.blogspot.com

Share this post